Triángulo

Este artículo trata sobre la forma geométrica básica. Para otros usos, consulte Triángulo (desambiguación) .

Shape with three sides

Triángulo equilátero
Un triangulo regular
Tipo	Polígono regular
Aristas y vértices	3
Símbolo de Schläfli	{3}
Diagrama de Coxeter
Grupo de simetría	Diedro (D 3 ), orden 2 × 3
Ángulo interno ( grados )	60 °
Polígono dual	Uno mismo
Propiedades	Convexo , cíclico , equilátero , isogonal , isotoxal

Triángulo
Un triángulo
Aristas y vértices	3
Símbolo de Schläfli	{3} (para equilátero)
Área	varios métodos; vea abajo
Ángulo interno ( grados )	60 ° (para equilátero)

Triángulo = Tri (tres) + Ángulo

Un triángulo es un polígono con tres aristas y tres vértices . Es una de las formas básicas en geometría . Un triángulo con vértices A , B , y C se denota . ^[1]${\displaystyle \triangle ABC}$

En la geometría euclidiana , tres puntos cualesquiera, cuando no son colineales , determinan un triángulo único y, simultáneamente, un plano único (es decir, un espacio euclidiano bidimensional ). En otras palabras, solo hay un plano que contiene ese triángulo, y cada triángulo está contenido en algún plano. Si toda la geometría es solo el plano euclidiano , solo hay un plano y todos los triángulos están contenidos en él; sin embargo, en los espacios euclidianos de dimensiones superiores, esto ya no es cierto. Este artículo trata sobre triángulos en geometría euclidiana y, en particular, el plano euclidiano, salvo que se indique lo contrario.

Tipos de triangulo

Diagrama de Euler de tipos de triángulos, utilizando la definición de que los triángulos isósceles tienen al menos 2 lados iguales (es decir, los triángulos equiláteros son isósceles).

La terminología para categorizar triángulos tiene más de dos mil años, habiendo sido definida en la primera página de los Elementos de Euclides . Los nombres utilizados para la clasificación moderna son una transliteración directa del griego de Euclides o sus traducciones latinas.

Por longitudes de lados

El matemático griego antiguo Euclides definió tres tipos de triángulos según la longitud de sus lados: ^{[2] [3]}

Griego : τῶν δὲ τριπλεύρων σχημάτων ἰσόπλευρον μὲν τρίγωνόν ἐστι τὸ τὰς τρεῖς ἴσας ἔχον πλευράς , ἰσοσκελὲς δὲ τὸ τὰς δύο μόνας ἴσας ἔχον πλευράς , σκαληνὸν δὲ τὸ τὰς τρεῖς ἀνίσους ἔχον πλευράς , lit.  "De las figuras trilaterales, un triángulo isopleuron [equilátero] es el que tiene sus tres lados iguales, un isósceles el que tiene dos de sus lados solamente iguales, y un escaleno el que tiene sus tres lados desiguales". ^[4]

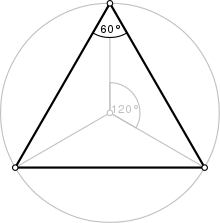
• Un triángulo equilátero ( griego : ἰσόπλευρον , romanizado :  isópleuron , literalmente  'lados iguales') tiene tres lados de la misma longitud. Un triángulo equilátero también es un polígono regular con todos los ángulos que miden 60 °. ^[5]
• Un triángulo isósceles ( griego : ἰσοσκελὲς , romanizado :  isoskelés , literalmente  , 'piernas iguales') tiene dos lados de igual longitud. ^{[nota 1] [6]} Un triángulo isósceles también tiene dos ángulos de la misma medida, es decir, los ángulos opuestos a los dos lados de la misma longitud. Este hecho es el contenido del teorema del triángulo isósceles , conocido por Euclides . Algunos matemáticos definen un triángulo isósceles para tener exactamente dos lados iguales, mientras que otros definen un triángulo isósceles como uno con al menos dos lados iguales. ^[6]La última definición haría que todos los triángulos equiláteros sean triángulos isósceles. El triángulo rectángulo 45–45–90, que aparece en el mosaico cuadrado de tetrakis , es isósceles.
• Un triángulo escaleno ( griego : σκαληνὸν , romanizado :  skalinón , literalmente  'desigual') tiene todos sus lados de diferentes longitudes. ^{[7] De manera} equivalente, tiene todos los ángulos de diferente medida.

• Triángulo equilátero

• Triángulo isósceles

• Triángulo escaleno

Las marcas de trama , también llamadas marcas de graduación, se utilizan en diagramas de triángulos y otras figuras geométricas para identificar lados de igual longitud. ^[1] Un lado se puede marcar con un patrón de "garrapatas", segmentos de línea cortos en forma de marcas de conteo ; dos lados tienen la misma longitud si ambos están marcados con el mismo patrón. En un triángulo, el patrón no suele tener más de 3 tics. Un triángulo equilátero tiene el mismo patrón en los 3 lados, un triángulo isósceles tiene el mismo patrón en solo 2 lados y un triángulo escaleno tiene diferentes patrones en todos los lados ya que ningún lado es igual.

De manera similar, los patrones de 1, 2 o 3 arcos concéntricos dentro de los ángulos se utilizan para indicar ángulos iguales: un triángulo equilátero tiene el mismo patrón en los 3 ángulos, un triángulo isósceles tiene el mismo patrón en solo 2 ángulos y un triángulo escaleno tiene diferentes patrones en todos los ángulos, ya que ningún ángulo es igual.

Por ángulos internos

La primera página de los Elementos de Euclides , de la primera versión impresa del mundo (1482), que muestra la sección de "definiciones" del Libro I. El triángulo rectángulo está etiquetado como " ortogonius ", y los dos ángulos que se muestran son "acutus" y "angulus obtusus". .

Los triángulos también se pueden clasificar según sus ángulos internos , medidos aquí en grados .

• Un triángulo rectángulo (o triángulo rectángulo , anteriormente llamado triángulo rectángulo ) tiene uno de sus ángulos interiores que mide 90 ° (un ángulo recto ). El lado opuesto al ángulo recto es la hipotenusa , el lado más largo del triángulo. Los otros dos lados se llaman catetos o cateti ^[8] (singular: cateto ) del triángulo. Los triángulos rectángulos obedecen al teorema de Pitágoras : la suma de los cuadrados de las longitudes de los dos catetos es igual al cuadrado de la longitud de la hipotenusa: a ² + b ² = c ², Donde un y b son las longitudes de las piernas y c es la longitud de la hipotenusa. Los triángulos rectángulos especiales son triángulos rectángulos con propiedades adicionales que facilitan los cálculos que los involucran. Uno de los dos más famosos es el triángulo rectángulo 3–4–5, donde 3 ² + 4 ² = 5 ² . En esta situación, 3, 4 y 5 son un triple pitagórico . El otro es un triángulo isósceles que tiene 2 ángulos que miden 45 grados (triángulo 45-45-90).
  • Los triángulos que no tienen un ángulo de 90 ° se llaman triángulos oblicuos .
• Un triángulo cuyos ángulos interiores miden menos de 90 ° es un triángulo agudo o un triángulo agudo . ^[3] Si c es la longitud del lado más largo, entonces a ² + b ² > c ² , donde a y b son las longitudes de los otros lados.
• Un triángulo con un ángulo interior que mide más de 90 ° es un triángulo obtuso o un triángulo de ángulo obtuso . ^[3] Si c es la longitud del lado más largo, entonces a ² + b ² < c ² , donde a y b son las longitudes de los otros lados.
• Un triángulo con un ángulo interior de 180 ° (y vértices colineales ) está degenerado . Un triángulo degenerado recto tiene vértices colineales, dos de los cuales son coincidentes.

Un triángulo que tiene dos ángulos con la misma medida también tiene dos lados con la misma longitud y, por lo tanto, es un triángulo isósceles. De ello se deduce que en un triángulo donde todos los ángulos tienen la misma medida, los tres lados tienen la misma longitud y, por lo tanto, es equilátero.

Derecha	Obtuso	Agudo
	${\displaystyle \underbrace {\qquad \qquad \qquad \qquad \qquad \qquad } _{}}$
	Oblicuo

Hechos básicos

Un triángulo que muestra el ángulo exterior d.

Se supone que los triángulos son figuras planas bidimensionales , a menos que el contexto indique lo contrario (consulte Triángulos no planos , a continuación). En tratamientos rigurosos, un triángulo se llama por lo tanto un 2- simplex (ver también Polytope ). Euclides presentó datos elementales sobre triángulos en los libros 1 a 4 de sus Elementos , escritos alrededor del año 300 a. C.

Las medidas de los ángulos interiores del triángulo siempre suman 180 grados (mismo color para señalar que son iguales).

La suma de las medidas de los ángulos interiores de un triángulo en el espacio euclidiano es siempre 180 grados. ^{[9] [3]} Este hecho es equivalente al postulado paralelo de Euclides . Esto permite determinar la medida del tercer ángulo de cualquier triángulo, dada la medida de dos ángulos. Un ángulo exterior de un triángulo es un ángulo que es un par lineal (y por lo tanto suplementario ) a un ángulo interior. La medida de un ángulo exterior de un triángulo es igual a la suma de las medidas de los dos ángulos interiores que no son adyacentes a él; este es el teorema del ángulo exterior. La suma de las medidas de los tres ángulos exteriores (uno para cada vértice) de cualquier triángulo es 360 grados. ^{[nota 2]}

Similitud y congruencia

Se dice que dos triángulos son similares , si cada ángulo de un triángulo tiene la misma medida que el ángulo correspondiente en el otro triángulo. Los lados correspondientes de triángulos similares tienen longitudes que están en la misma proporción, y esta propiedad también es suficiente para establecer similitudes.

Algunos teoremas básicos sobre triángulos semejantes son:

• Si y solo si un par de ángulos internos de dos triángulos tienen la misma medida que el otro, y otro par también tiene la misma medida que el otro, los triángulos son similares.
• Si y solo si un par de lados correspondientes de dos triángulos están en la misma proporción que otro par de lados correspondientes, y sus ángulos incluidos tienen la misma medida, entonces los triángulos son similares. (El ángulo incluido para dos lados cualesquiera de un polígono es el ángulo interno entre esos dos lados).
• Si y solo si tres pares de lados correspondientes de dos triángulos están todos en la misma proporción, entonces los triángulos son similares. ^{[nota 3]}

Dos triángulos que son congruentes tienen exactamente el mismo tamaño y forma: ^{[nota 4]} todos los pares de ángulos interiores correspondientes son iguales en medida, y todos los pares de lados correspondientes tienen la misma longitud. (Esto es un total de seis igualdades, pero tres suelen ser suficientes para demostrar la congruencia).

Algunas condiciones individualmente necesarias y suficientes para que un par de triángulos sea congruente son:

• Postulado SAS: Dos lados de un triángulo tienen la misma longitud que dos lados del otro triángulo, y los ángulos incluidos tienen la misma medida.
• ASA: Dos ángulos interiores y el lado incluido en un triángulo tienen la misma medida y longitud, respectivamente, que los del otro triángulo. (El lado incluido para un par de ángulos es el lado que les es común).
• SSS: Cada lado de un triángulo tiene la misma longitud que el lado correspondiente del otro triángulo.
• AAS: Dos ángulos y un lado correspondiente (no incluido) en un triángulo tienen la misma medida y longitud, respectivamente, que los del otro triángulo. (Esto a veces se denomina AAcorrS y luego incluye ASA arriba).

Algunas condiciones individuales suficientes son:

• Teorema de hipotenusa-cateto (HL): La hipotenusa y un cateto de un triángulo rectángulo tienen la misma longitud que los de otro triángulo rectángulo. Esto también se llama RHS (ángulo recto, hipotenusa, lado).
• Teorema de hipotenusa-ángulo: la hipotenusa y un ángulo agudo en un triángulo rectángulo tienen la misma longitud y medida, respectivamente, que los del otro triángulo rectángulo. Este es solo un caso particular del teorema de AAS.

Una condición importante es:

• Condición de lado-lado-ángulo (o ángulo-lado-lado): si dos lados y un ángulo no incluido correspondiente de un triángulo tienen la misma longitud y medida, respectivamente, que los de otro triángulo, entonces esto no es suficiente para demostrar congruencia; pero si el ángulo dado es opuesto al lado más largo de los dos lados, entonces los triángulos son congruentes. El teorema hipotenusa-pierna es un caso particular de este criterio. La condición Side-Side-Angle no garantiza por sí misma que los triángulos sean congruentes porque un triángulo podría tener un ángulo obtuso y el otro un ángulo agudo.

Usando triángulos rectángulos y el concepto de semejanza, se pueden definir las funciones trigonométricas seno y coseno. Estas son funciones de un ángulo que se investigan en trigonometría .

Triángulos rectángulos

El teorema de Pitágoras

Un teorema central es el teorema de Pitágoras , que establece en cualquier triángulo rectángulo , el cuadrado de la longitud de la hipotenusa es igual a la suma de los cuadrados de las longitudes de los otros dos lados. Si la hipotenusa tiene una longitud c , y las piernas tienen longitudes a y b , entonces los estados teorema que

${\displaystyle a^{2}+b^{2}=c^{2}.}$

Lo contrario es cierto: si las longitudes de los lados de un triángulo satisfacen la ecuación anterior, entonces el triángulo tiene un ángulo recto opuesto al lado c .

Algunos otros datos sobre los triángulos rectángulos:

• Los ángulos agudos de un triángulo rectángulo son complementarios .

${\displaystyle a+b+90^{\circ }=180^{\circ }\Rightarrow a+b=90^{\circ }\Rightarrow a=90^{\circ }-b.}$

• Si los catetos de un triángulo rectángulo tienen la misma longitud, entonces los ángulos opuestos a esos catetos tienen la misma medida. Dado que estos ángulos son complementarios, se deduce que cada uno mide 45 grados. Según el teorema de Pitágoras, la longitud de la hipotenusa es la longitud de un cateto multiplicado por √ 2 .
• En un triángulo rectángulo con ángulos agudos que miden 30 y 60 grados, la hipotenusa es el doble de la longitud del lado más corto y el lado más largo es igual a la longitud del lado más corto multiplicado por √ 3 :

${\displaystyle c=2a\,}$

${\displaystyle b=a\times {\sqrt {3}}.}$

Para todos los triángulos, los ángulos y los lados están relacionados por la ley de los cosenos y la ley de los senos (también llamada regla del coseno y regla del seno ).

Existencia de un triángulo

Condición en los lados

La desigualdad del triángulo establece que la suma de las longitudes de dos lados cualesquiera de un triángulo debe ser mayor o igual que la longitud del tercer lado. Esa suma puede ser igual a la longitud del tercer lado solo en el caso de un triángulo degenerado, uno con vértices colineales. No es posible que esa suma sea menor que la longitud del tercer lado. Un triángulo con tres longitudes de lados positivas dadas existe si y solo si esas longitudes de lados satisfacen la desigualdad del triángulo.

Condiciones en los ángulos

Tres ángulos dados forman un triángulo no degenerado (y de hecho una infinitud de ellos) si y solo si se cumplen ambas condiciones: (a) cada uno de los ángulos es positivo, y (b) los ángulos suman 180 °. Si se permiten triángulos degenerados, se permiten ángulos de 0 °.

Condiciones trigonométricas

Tres ángulos positivos α , β y γ , cada uno de ellos menor de 180 °, son los ángulos de un triángulo si y solo si se cumple alguna de las siguientes condiciones:

${\displaystyle \tan {\frac {\alpha }{2}}\tan {\frac {\beta }{2}}+\tan {\frac {\beta }{2}}\tan {\frac {\gamma }{2}}+\tan {\frac {\gamma }{2}}\tan {\frac {\alpha }{2}}=1,}$^[10]

${\displaystyle \sin ^{2}{\frac {\alpha }{2}}+\sin ^{2}{\frac {\beta }{2}}+\sin ^{2}{\frac {\gamma }{2}}+2\sin {\frac {\alpha }{2}}\sin {\frac {\beta }{2}}\sin {\frac {\gamma }{2}}=1,}$^[10]

${\displaystyle \sin(2\alpha )+\sin(2\beta )+\sin(2\gamma )=4\sin(\alpha )\sin(\beta )\sin(\gamma ),}$

${\displaystyle \cos ^{2}\alpha +\cos ^{2}\beta +\cos ^{2}\gamma +2\cos(\alpha )\cos(\beta )\cos(\gamma )=1,}$^[11]

${\displaystyle \tan(\alpha )+\tan(\beta )+\tan(\gamma )=\tan(\alpha )\tan(\beta )\tan(\gamma ),}$

la última igualdad se aplica solo si ninguno de los ángulos es de 90 ° (por lo que el valor de la función tangente es siempre finito).

Puntos, líneas y círculos asociados con un triángulo

Hay miles de construcciones diferentes que encuentran un punto especial asociado con (y a menudo dentro) de un triángulo, satisfaciendo alguna propiedad única: consulte el artículo Enciclopedia de centros de triángulos para obtener un catálogo de ellas. A menudo se construyen encontrando tres líneas asociadas de manera simétrica con los tres lados (o vértices) y luego probando que las tres líneas se encuentran en un solo punto: una herramienta importante para probar la existencia de estas es el teorema de Ceva , que da criterio para determinar cuándo tres de estas líneas son concurrentes . De manera similar, las líneas asociadas con un triángulo a menudo se construyen demostrando que tres puntos construidos simétricamente son colineales : aquí el teorema de Menelaoda un criterio general útil. En esta sección se explican solo algunas de las construcciones más comunes.

El circuncentro es el centro de un círculo que pasa por los tres vértices del triángulo.

Una bisectriz perpendicular de un lado de un triángulo es una línea recta que pasa por el punto medio del lado y es perpendicular a él, es decir, forma un ángulo recto con él. Las tres bisectrices perpendiculares se encuentran en un solo punto, el circuncentro del triángulo , generalmente denotado por O ; este punto es el centro de la circunferencia , el círculo que pasa por los tres vértices. El diámetro de este círculo, llamado perímetro , se puede encontrar a partir de la ley de los senos indicada anteriormente. El radio de la circunferencia se llama circunferencia .

El teorema de Tales implica que si el circuncentro está ubicado en un lado del triángulo, entonces el ángulo opuesto es recto. Si el circuncentro está ubicado dentro del triángulo, entonces el triángulo es agudo; si el circuncentro está ubicado fuera del triángulo, entonces el triángulo es obtuso.

La intersección de las altitudes es el ortocentro .

La altura de un triángulo es una línea recta que pasa por un vértice y es perpendicular (es decir, forma un ángulo recto con) el lado opuesto. Este lado opuesto se llama la base de la altitud, y el punto donde la altitud se cruza con la base (o su extensión) se llama el pie de la altitud. La longitud de la altitud es la distancia entre la base y el vértice. Los tres altitudes se intersecan en un solo punto, llamado el ortocentro del triángulo, generalmente denotado por H . El ortocentro se encuentra dentro del triángulo si y solo si el triángulo es agudo.

La intersección de las bisectrices de los ángulos es el centro del círculo .

Una bisectriz de ángulo de un triángulo es una línea recta que pasa por un vértice que corta el ángulo correspondiente por la mitad. Las tres bisectrices de los ángulos se cruzan en un solo punto, el incentro , generalmente denotado por I , el centro del círculo del triángulo . El círculo es el círculo que se encuentra dentro del triángulo y toca los tres lados. Su radio se llama inradius . Hay otros tres círculos importantes, los excircles ; se encuentran fuera del triángulo y tocan un lado así como las extensiones de los otros dos. Los centros de los círculos internos y externos forman un sistema ortocéntrico .

La intersección de las medianas es el centroide .

La mediana de un triángulo es una línea recta que pasa por un vértice y el punto medio del lado opuesto, y divide el triángulo en dos áreas iguales. Las tres medianas de intersección en un solo punto, del triángulo centroide baricentro o geométrica, generalmente denotados por G . El centroide de un objeto triangular rígido (cortado de una hoja delgada de densidad uniforme) es también su centro de masa : el objeto puede equilibrarse sobre su centroide en un campo gravitacional uniforme. El centroide corta cada mediana en la proporción 2: 1, es decir, la distancia entre un vértice y el centroide es el doble de la distancia entre el centroide y el punto medio del lado opuesto.

Nine-point circle demonstrates a symmetry where six points lie on the edge of the triangle.

The midpoints of the three sides and the feet of the three altitudes all lie on a single circle, the triangle's nine-point circle. The remaining three points for which it is named are the midpoints of the portion of altitude between the vertices and the orthocenter. The radius of the nine-point circle is half that of the circumcircle. It touches the incircle (at the Feuerbach point) and the three excircles.

Euler's line is a straight line through the orthocenter (blue), center of the nine-point circle (red), centroid (orange), and circumcenter (green)

The orthocenter (blue point), center of the nine-point circle (red), centroid (orange), and circumcenter (green) all lie on a single line, known as Euler's line (red line). The center of the nine-point circle lies at the midpoint between the orthocenter and the circumcenter, and the distance between the centroid and the circumcenter is half that between the centroid and the orthocenter.

The center of the incircle is not in general located on Euler's line.

If one reflects a median in the angle bisector that passes through the same vertex, one obtains a symmedian. The three symmedians intersect in a single point, the symmedian point of the triangle.

Computing the sides and angles

There are various standard methods for calculating the length of a side or the measure of an angle. Certain methods are suited to calculating values in a right-angled triangle; more complex methods may be required in other situations.

Trigonometric ratios in right triangles

Main article: Trigonometric functions

A right triangle always includes a 90° (π/2 radians) angle, here with label C. Angles A and B may vary. Trigonometric functions specify the relationships among side lengths and interior angles of a right triangle.

In right triangles, the trigonometric ratios of sine, cosine and tangent can be used to find unknown angles and the lengths of unknown sides. The sides of the triangle are known as follows:

• The hypotenuse is the side opposite the right angle, or defined as the longest side of a right-angled triangle, in this case h.
• The opposite side is the side opposite to the angle we are interested in, in this case a.
• The adjacent side is the side that is in contact with the angle we are interested in and the right angle, hence its name. In this case the adjacent side is b.

Sine, cosine and tangent

The sine of an angle is the ratio of the length of the opposite side to the length of the hypotenuse. In our case

${\displaystyle \sin A={\frac {\text{opposite side}}{\text{hypotenuse}}}={\frac {a}{h}}\,.}$

This ratio does not depend on the particular right triangle chosen, as long as it contains the angle A, since all those triangles are similar.

The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse. In our case

${\displaystyle \cos A={\frac {\text{adjacent side}}{\text{hypotenuse}}}={\frac {b}{h}}\,.}$

The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side. In our case

${\displaystyle \tan A={\frac {\text{opposite side}}{\text{adjacent side}}}={\frac {a}{b}}={\frac {\sin A}{\cos A}}\,.}$

The acronym "SOH-CAH-TOA" is a useful mnemonic for these ratios.

Inverse functions

The inverse trigonometric functions can be used to calculate the internal angles for a right angled triangle with the length of any two sides.

Arcsin can be used to calculate an angle from the length of the opposite side and the length of the hypotenuse.

${\displaystyle \theta =\arcsin \left({\frac {\text{opposite side}}{\text{hypotenuse}}}\right)}$

Arccos can be used to calculate an angle from the length of the adjacent side and the length of the hypotenuse.

${\displaystyle \theta =\arccos \left({\frac {\text{adjacent side}}{\text{hypotenuse}}}\right)}$

Arctan can be used to calculate an angle from the length of the opposite side and the length of the adjacent side.

${\displaystyle \theta =\arctan \left({\frac {\text{opposite side}}{\text{adjacent side}}}\right)}$

In introductory geometry and trigonometry courses, the notation sin⁻¹, cos⁻¹, etc., are often used in place of arcsin, arccos, etc. However, the arcsin, arccos, etc., notation is standard in higher mathematics where trigonometric functions are commonly raised to powers, as this avoids confusion between multiplicative inverse and compositional inverse.

Sine, cosine and tangent rules

Main articles: Law of sines, Law of cosines, and Law of tangents

A triangle with sides of length a, b and c and angles of α, β and γ respectively.

The law of sines, or sine rule,^[12] states that the ratio of the length of a side to the sine of its corresponding opposite angle is constant, that is

${\displaystyle {\frac {a}{\sin \alpha }}={\frac {b}{\sin \beta }}={\frac {c}{\sin \gamma }}.}$

This ratio is equal to the diameter of the circumscribed circle of the given triangle. Another interpretation of this theorem is that every triangle with angles α, β and γ is similar to a triangle with side lengths equal to sin α, sin β and sin γ. This triangle can be constructed by first constructing a circle of diameter 1, and inscribing in it two of the angles of the triangle. The length of the sides of that triangle will be sin α, sin β and sin γ. The side whose length is sin α is opposite to the angle whose measure is α, etc.

The law of cosines, or cosine rule, connects the length of an unknown side of a triangle to the length of the other sides and the angle opposite to the unknown side.^[12] As per the law:

For a triangle with length of sides a, b, c and angles of α, β, γ respectively, given two known lengths of a triangle a and b, and the angle between the two known sides γ (or the angle opposite to the unknown side c), to calculate the third side c, the following formula can be used:

${\displaystyle c^{2}\ =a^{2}+b^{2}-2ab\cos(\gamma )}$

${\displaystyle b^{2}\ =a^{2}+c^{2}-2ac\cos(\beta )}$

${\displaystyle a^{2}\ =b^{2}+c^{2}-2bc\cos(\alpha )}$

If the lengths of all three sides of any triangle are known the three angles can be calculated:

${\displaystyle \alpha =\arccos \left({\frac {b^{2}+c^{2}-a^{2}}{2bc}}\right)}$

${\displaystyle \beta =\arccos \left({\frac {a^{2}+c^{2}-b^{2}}{2ac}}\right)}$

${\displaystyle \gamma =\arccos \left({\frac {a^{2}+b^{2}-c^{2}}{2ab}}\right)}$

The law of tangents, or tangent rule, can be used to find a side or an angle when two sides and an angle or two angles and a side are known. It states that:^[13]

${\displaystyle {\frac {a-b}{a+b}}={\frac {\tan[{\frac {1}{2}}(\alpha -\beta )]}{\tan[{\frac {1}{2}}(\alpha +\beta )]}}.}$

Solution of triangles

Main article: Solution of triangles

"Solution of triangles" is the main trigonometric problem: to find missing characteristics of a triangle (three angles, the lengths of the three sides etc.) when at least three of these characteristics are given. The triangle can be located on a plane or on a sphere. This problem often occurs in various trigonometric applications, such as geodesy, astronomy, construction, navigation etc.

Computing the area of a triangle

The area of a triangle can be demonstrated, for example by means of the congruence of triangles, as half of the area of a parallelogram that has the same base length and height.

A graphic derivation of the formula ${\displaystyle T={\frac {h}{2}}b}$ that avoids the usual procedure of doubling the area of the triangle and then halving it.

See also: Congruence (geometry) § Congruence of triangles

Calculating the area T of a triangle is an elementary problem encountered often in many different situations. The best known and simplest formula is:

${\displaystyle T={\frac {1}{2}}bh,}$

where b is the length of the base of the triangle, and h is the height or altitude of the triangle. The term "base" denotes any side, and "height" denotes the length of a perpendicular from the vertex opposite the base onto the line containing the base. In 499 CE Aryabhata, used this illustrated method in the Aryabhatiya (section 2.6).^[14]

Although simple, this formula is only useful if the height can be readily found, which is not always the case. For example, the surveyor of a triangular field might find it relatively easy to measure the length of each side, but relatively difficult to construct a 'height'. Various methods may be used in practice, depending on what is known about the triangle. The following is a selection of frequently used formulae for the area of a triangle.^[15]

Using trigonometry

Applying trigonometry to find the altitude h.

The height of a triangle can be found through the application of trigonometry.

Knowing SAS: Using the labels in the image on the right, the altitude is h = a sin ${\displaystyle \gamma }$. Substituting this in the formula ${\displaystyle T={\frac {1}{2}}bh}$ derived above, the area of the triangle can be expressed as:

${\displaystyle T={\frac {1}{2}}ab\sin \gamma ={\frac {1}{2}}bc\sin \alpha ={\frac {1}{2}}ca\sin \beta }$

(where α is the interior angle at A, β is the interior angle at B, ${\displaystyle \gamma }$ is the interior angle at C and c is the line AB).

Furthermore, since sin α = sin (π − α) = sin (β + ${\displaystyle \gamma }$), and similarly for the other two angles:

${\displaystyle T={\frac {1}{2}}ab\sin(\alpha +\beta )={\frac {1}{2}}bc\sin(\beta +\gamma )={\frac {1}{2}}ca\sin(\gamma +\alpha ).}$

Knowing AAS:

${\displaystyle T={\frac {b^{2}(\sin \alpha )(\sin(\alpha +\beta ))}{2\sin \beta }},}$

and analogously if the known side is a or c.

Knowing ASA:^[2]

${\displaystyle T={\frac {a^{2}}{2(\cot \beta +\cot \gamma )}}={\frac {a^{2}(\sin \beta )(\sin \gamma )}{2\sin(\beta +\gamma )}},}$

and analogously if the known side is b or c.

Using Heron's formula

The shape of the triangle is determined by the lengths of the sides. Therefore, the area can also be derived from the lengths of the sides. By Heron's formula:

${\displaystyle T={\sqrt {s(s-a)(s-b)(s-c)}}}$

where ${\displaystyle s={\tfrac {a+b+c}{2}}}$ is the semiperimeter, or half of the triangle's perimeter.

Three other equivalent ways of writing Heron's formula are

${\displaystyle T={\frac {1}{4}}{\sqrt {(a^{2}+b^{2}+c^{2})^{2}-2(a^{4}+b^{4}+c^{4})}}}$

${\displaystyle T={\frac {1}{4}}{\sqrt {2(a^{2}b^{2}+a^{2}c^{2}+b^{2}c^{2})-(a^{4}+b^{4}+c^{4})}}}$

${\displaystyle T={\frac {1}{4}}{\sqrt {(a+b-c)(a-b+c)(-a+b+c)(a+b+c)}}.}$

Using vectors

The area of a parallelogram embedded in a three-dimensional Euclidean space can be calculated using vectors. Let vectors AB and AC point respectively from A to B and from A to C. The area of parallelogram ABDC is then

${\displaystyle |\mathbf {AB} \times \mathbf {AC} |,}$

which is the magnitude of the cross product of vectors AB and AC. The area of triangle ABC is half of this,

${\displaystyle {\frac {1}{2}}|\mathbf {AB} \times \mathbf {AC} |.}$

The area of triangle ABC can also be expressed in terms of dot products as follows:

${\displaystyle {\frac {1}{2}}{\sqrt {(\mathbf {AB} \cdot \mathbf {AB} )(\mathbf {AC} \cdot \mathbf {AC} )-(\mathbf {AB} \cdot \mathbf {AC} )^{2}}}={\frac {1}{2}}{\sqrt {|\mathbf {AB} |^{2}|\mathbf {AC} |^{2}-(\mathbf {AB} \cdot \mathbf {AC} )^{2}}}.\,}$

In two-dimensional Euclidean space, expressing vector AB as a free vector in Cartesian space equal to (x₁,y₁) and AC as (x₂,y₂), this can be rewritten as:

${\displaystyle {\frac {1}{2}}\,|x_{1}y_{2}-x_{2}y_{1}|.\,}$

Using coordinates

If vertex A is located at the origin (0, 0) of a Cartesian coordinate system and the coordinates of the other two vertices are given by B = (x_B, y_B) and C = (x_C, y_C), then the area can be computed as 1⁄2 times the absolute value of the determinant

${\displaystyle T={\frac {1}{2}}\left|\det {\begin{pmatrix}x_{B}&x_{C}\\y_{B}&y_{C}\end{pmatrix}}\right|={\frac {1}{2}}|x_{B}y_{C}-x_{C}y_{B}|.}$

For three general vertices, the equation is:

${\displaystyle T={\frac {1}{2}}\left|\det {\begin{pmatrix}x_{A}&x_{B}&x_{C}\\y_{A}&y_{B}&y_{C}\\1&1&1\end{pmatrix}}\right|={\frac {1}{2}}{\big |}x_{A}y_{B}-x_{A}y_{C}+x_{B}y_{C}-x_{B}y_{A}+x_{C}y_{A}-x_{C}y_{B}{\big |},}$

which can be written as

${\displaystyle T={\frac {1}{2}}{\big |}(x_{A}-x_{C})(y_{B}-y_{A})-(x_{A}-x_{B})(y_{C}-y_{A}){\big |}.}$

If the points are labeled sequentially in the counterclockwise direction, the above determinant expressions are positive and the absolute value signs can be omitted.^[16] The above formula is known as the shoelace formula or the surveyor's formula.

If we locate the vertices in the complex plane and denote them in counterclockwise sequence as a = x_A + y_Ai, b = x_B + y_Bi, and c = x_C + y_Ci, and denote their complex conjugates as ${\displaystyle {\bar {a}}}$, ${\displaystyle {\bar {b}}}$, and ${\displaystyle {\bar {c}}}$, then the formula

${\displaystyle T={\frac {i}{4}}{\begin{vmatrix}a&{\bar {a}}&1\\b&{\bar {b}}&1\\c&{\bar {c}}&1\end{vmatrix}}}$

is equivalent to the shoelace formula.

In three dimensions, the area of a general triangle A = (x_A, y_A, z_A), B = (x_B, y_B, z_B) and C = (x_C, y_C, z_C) is the Pythagorean sum of the areas of the respective projections on the three principal planes (i.e. x = 0, y = 0 and z = 0):

${\displaystyle T={\frac {1}{2}}{\sqrt {{\begin{vmatrix}x_{A}&x_{B}&x_{C}\\y_{A}&y_{B}&y_{C}\\1&1&1\end{vmatrix}}^{2}+{\begin{vmatrix}y_{A}&y_{B}&y_{C}\\z_{A}&z_{B}&z_{C}\\1&1&1\end{vmatrix}}^{2}+{\begin{vmatrix}z_{A}&z_{B}&z_{C}\\x_{A}&x_{B}&x_{C}\\1&1&1\end{vmatrix}}^{2}}}.}$

Using line integrals

The area within any closed curve, such as a triangle, is given by the line integral around the curve of the algebraic or signed distance of a point on the curve from an arbitrary oriented straight line L. Points to the right of L as oriented are taken to be at negative distance from L, while the weight for the integral is taken to be the component of arc length parallel to L rather than arc length itself.

This method is well suited to computation of the area of an arbitrary polygon. Taking L to be the x-axis, the line integral between consecutive vertices (x_i,y_i) and (x_i+1,y_i+1) is given by the base times the mean height, namely (x_i+1 − x_i)(y_i + y_i+1)/2. The sign of the area is an overall indicator of the direction of traversal, with negative area indicating counterclockwise traversal. The area of a triangle then falls out as the case of a polygon with three sides.

While the line integral method has in common with other coordinate-based methods the arbitrary choice of a coordinate system, unlike the others it makes no arbitrary choice of vertex of the triangle as origin or of side as base. Furthermore, the choice of coordinate system defined by L commits to only two degrees of freedom rather than the usual three, since the weight is a local distance (e.g. x_i+1 − x_i in the above) whence the method does not require choosing an axis normal to L.

When working in polar coordinates it is not necessary to convert to Cartesian coordinates to use line integration, since the line integral between consecutive vertices (r_i,θ_i) and (r_i+1,θ_i+1) of a polygon is given directly by r_ir_i+1sin(θ_i+1 − θ_i)/2. This is valid for all values of θ, with some decrease in numerical accuracy when |θ| is many orders of magnitude greater than π. With this formulation negative area indicates clockwise traversal, which should be kept in mind when mixing polar and cartesian coordinates. Just as the choice of y-axis (x = 0) is immaterial for line integration in cartesian coordinates, so is the choice of zero heading (θ = 0) immaterial here.

Formulas resembling Heron's formula

Three formulas have the same structure as Heron's formula but are expressed in terms of different variables. First, denoting the medians from sides a, b, and c respectively as m_a, m_b, and m_c and their semi-sum (m_a + m_b + m_c)/2 as σ, we have^[17]

${\displaystyle T={\frac {4}{3}}{\sqrt {\sigma (\sigma -m_{a})(\sigma -m_{b})(\sigma -m_{c})}}.}$

Next, denoting the altitudes from sides a, b, and c respectively as h_a, h_b, and h_c, and denoting the semi-sum of the reciprocals of the altitudes as ${\displaystyle H=(h_{a}^{-1}+h_{b}^{-1}+h_{c}^{-1})/2}$ we have^[18]

${\displaystyle T^{-1}=4{\sqrt {H(H-h_{a}^{-1})(H-h_{b}^{-1})(H-h_{c}^{-1})}}.}$

And denoting the semi-sum of the angles' sines as S = [(sin α) + (sin β) + (sin γ)]/2, we have^[19]

${\displaystyle T=D^{2}{\sqrt {S(S-\sin \alpha )(S-\sin \beta )(S-\sin \gamma )}}}$

where D is the diameter of the circumcircle: ${\displaystyle D={\tfrac {a}{\sin \alpha }}={\tfrac {b}{\sin \beta }}={\tfrac {c}{\sin \gamma }}.}$

Using Pick's theorem

See Pick's theorem for a technique for finding the area of any arbitrary lattice polygon (one drawn on a grid with vertically and horizontally adjacent lattice points at equal distances, and with vertices on lattice points).

The theorem states:

${\displaystyle T=I+{\frac {1}{2}}B-1}$

where ${\displaystyle I}$ is the number of internal lattice points and B is the number of lattice points lying on the border of the polygon.

Other area formulas

Numerous other area formulas exist, such as

${\displaystyle T=r\cdot s,}$

where r is the inradius, and s is the semiperimeter (in fact, this formula holds for all tangential polygons), and^{[20]:Lemma 2}

${\displaystyle T=r_{a}(s-a)=r_{b}(s-b)=r_{c}(s-c)}$

where ${\displaystyle r_{a},\,r_{b},\,r_{c}}$ are the radii of the excircles tangent to sides a, b, c respectively.

We also have

${\displaystyle T={\frac {1}{2}}D^{2}(\sin \alpha )(\sin \beta )(\sin \gamma )}$

and^[21]

${\displaystyle T={\frac {abc}{2D}}={\frac {abc}{4R}}}$

for circumdiameter D; and^[22]

${\displaystyle T={\frac {\tan \alpha }{4}}(b^{2}+c^{2}-a^{2})}$

for angle α ≠ 90°.

The area can also be expressed as^[23]

${\displaystyle T={\sqrt {rr_{a}r_{b}r_{c}}}.}$

In 1885, Baker^[24] gave a collection of over a hundred distinct area formulas for the triangle. These include:

${\displaystyle T={\frac {1}{2}}[abch_{a}h_{b}h_{c}]^{1/3},}$

${\displaystyle T={\frac {1}{2}}{\sqrt {abh_{a}h_{b}}},}$

${\displaystyle T={\frac {a+b}{2(h_{a}^{-1}+h_{b}^{-1})}},}$

${\displaystyle T={\frac {Rh_{b}h_{c}}{a}}}$

for circumradius (radius of the circumcircle) R, and

${\displaystyle T={\frac {h_{a}h_{b}}{2\sin \gamma }}.}$

Upper bound on the area

The area T of any triangle with perimeter p satisfies

${\displaystyle T\leq {\tfrac {p^{2}}{12{\sqrt {3}}}},}$

with equality holding if and only if the triangle is equilateral.^[25][26]:657

Other upper bounds on the area T are given by^[27]:p.290

${\displaystyle 4{\sqrt {3}}T\leq a^{2}+b^{2}+c^{2}}$

and

${\displaystyle 4{\sqrt {3}}T\leq {\frac {9abc}{a+b+c}},}$

both again holding if and only if the triangle is equilateral.

Bisecting the area

There are infinitely many lines that bisect the area of a triangle.^[28] Three of them are the medians, which are the only area bisectors that go through the centroid. Three other area bisectors are parallel to the triangle's sides.

Any line through a triangle that splits both the triangle's area and its perimeter in half goes through the triangle's incenter. There can be one, two, or three of these for any given triangle.

Further formulas for general Euclidean triangles

See also: List of triangle inequalities

The formulas in this section are true for all Euclidean triangles.

Medians, angle bisectors, perpendicular side bisectors, and altitudes

Main articles: Median (geometry), Angle bisector, Bisection § Perpendicular bisectors, and Altitude (geometry)

The medians and the sides are related by^[29]:p.70

${\displaystyle {\frac {3}{4}}(a^{2}+b^{2}+c^{2})=m_{a}^{2}+m_{b}^{2}+m_{c}^{2}}$

and

${\displaystyle m_{a}={\frac {1}{2}}{\sqrt {2b^{2}+2c^{2}-a^{2}}}={\sqrt {{\frac {1}{2}}(a^{2}+b^{2}+c^{2})-{\frac {3}{4}}a^{2}}}}$,

and equivalently for m_b and m_c.

For angle A opposite side a, the length of the internal angle bisector is given by^[30]

${\displaystyle w_{A}={\frac {2{\sqrt {bcs(s-a)}}}{b+c}}={\sqrt {bc\left[1-{\frac {a^{2}}{(b+c)^{2}}}\right]}}={\frac {2bc}{b+c}}\cos {\frac {A}{2}},}$

for semiperimeter s, where the bisector length is measured from the vertex to where it meets the opposite side.

The interior perpendicular bisectors are given by

${\displaystyle p_{a}={\frac {2aT}{a^{2}+b^{2}-c^{2}}},}$

${\displaystyle p_{b}={\frac {2bT}{a^{2}+b^{2}-c^{2}}},}$

${\displaystyle p_{c}={\frac {2cT}{a^{2}-b^{2}+c^{2}}},}$

where the sides are ${\displaystyle a\geq b\geq c}$ and the area is ${\displaystyle T.}$^{[31]:Thm 2}

The altitude from, for example, the side of length a is

${\displaystyle h_{a}={\frac {2T}{a}}.}$

Circumradius and inradius

Main articles: Circumradius and Inradius

The following formulas involve the circumradius R and the inradius r:

${\displaystyle R={\sqrt {\frac {a^{2}b^{2}c^{2}}{(a+b+c)(-a+b+c)(a-b+c)(a+b-c)}}};}$

${\displaystyle r={\sqrt {\frac {(-a+b+c)(a-b+c)(a+b-c)}{4(a+b+c)}}};}$

${\displaystyle {\frac {1}{r}}={\frac {1}{h_{a}}}+{\frac {1}{h_{b}}}+{\frac {1}{h_{c}}}}$

where h_a etc. are the altitudes to the subscripted sides;^[29]:p.79

${\displaystyle {\frac {r}{R}}={\frac {4T^{2}}{sabc}}=\cos \alpha +\cos \beta +\cos \gamma -1;}$^[11]

and

${\displaystyle 2Rr={\frac {abc}{a+b+c}}}$.

The product of two sides of a triangle equals the altitude to the third side times the diameter D of the circumcircle:^[29]:p.64

${\displaystyle ab=h_{c}D,\quad \quad bc=h_{a}D,\quad ca=h_{b}D.}$

Adjacent triangles

Suppose two adjacent but non-overlapping triangles share the same side of length f and share the same circumcircle, so that the side of length f is a chord of the circumcircle and the triangles have side lengths (a, b, f) and (c, d, f), with the two triangles together forming a cyclic quadrilateral with side lengths in sequence (a, b, c, d). Then^[32]:84

${\displaystyle f^{2}={\frac {(ac+bd)(ad+bc)}{(ab+cd)}}.\,}$

Centroid

Main article: Centroid

Let G be the centroid of a triangle with vertices A, B, and C, and let P be any interior point. Then the distances between the points are related by^[32]:174

${\displaystyle (PA)^{2}+(PB)^{2}+(PC)^{2}=(GA)^{2}+(GB)^{2}+(GC)^{2}+3(PG)^{2}.\,}$

The sum of the squares of the triangle's sides equals three times the sum of the squared distances of the centroid from the vertices:

${\displaystyle AB^{2}+BC^{2}+CA^{2}=3(GA^{2}+GB^{2}+GC^{2}).}$^[33]

Let q_a, q_b, and q_c be the distances from the centroid to the sides of lengths a, b, and c. Then^[32]:173

${\displaystyle {\frac {q_{a}}{q_{b}}}={\frac {b}{a}},\quad \quad {\frac {q_{b}}{q_{c}}}={\frac {c}{b}},\quad \quad {\frac {q_{a}}{q_{c}}}={\frac {c}{a}}\,}$

and

${\displaystyle q_{a}\cdot a=q_{b}\cdot b=q_{c}\cdot c={\frac {2}{3}}T\,}$

for area T.

Circumcenter, incenter, and orthocenter

Main articles: Circumcenter, Incenter, and Orthocenter

Carnot's theorem states that the sum of the distances from the circumcenter to the three sides equals the sum of the circumradius and the inradius.^[29]:p.83 Here a segment's length is considered to be negative if and only if the segment lies entirely outside the triangle. This method is especially useful for deducing the properties of more abstract forms of triangles, such as the ones induced by Lie algebras, that otherwise have the same properties as usual triangles.

Euler's theorem states that the distance d between the circumcenter and the incenter is given by^[29]:p.85

${\displaystyle \displaystyle d^{2}=R(R-2r)}$

or equivalently

${\displaystyle {\frac {1}{R-d}}+{\frac {1}{R+d}}={\frac {1}{r}},}$

where R is the circumradius and r is the inradius. Thus for all triangles R ≥ 2r, with equality holding for equilateral triangles.

If we denote that the orthocenter divides one altitude into segments of lengths u and v, another altitude into segment lengths w and x, and the third altitude into segment lengths y and z, then uv = wx = yz.^[29]:p.94

The distance from a side to the circumcenter equals half the distance from the opposite vertex to the orthocenter.^[29]:p.99

The sum of the squares of the distances from the vertices to the orthocenter H plus the sum of the squares of the sides equals twelve times the square of the circumradius:^[29]:p.102

${\displaystyle AH^{2}+BH^{2}+CH^{2}+a^{2}+b^{2}+c^{2}=12R^{2}.}$

Angles

In addition to the law of sines, the law of cosines, the law of tangents, and the trigonometric existence conditions given earlier, for any triangle

${\displaystyle a=b\cos C+c\cos B,\quad b=c\cos A+a\cos C,\quad c=a\cos B+b\cos A.}$

Morley's trisector theorem

Main article: Morley's trisector theorem

The Morley triangle, resulting from the trisection of each interior angle. This is an example of a finite subdivision rule.

Morley's trisector theorem states that in any triangle, the three points of intersection of the adjacent angle trisectors form an equilateral triangle, called the Morley triangle.

Figures inscribed in a triangle

Conics

As discussed above, every triangle has a unique inscribed circle (incircle) that is interior to the triangle and tangent to all three sides.

Every triangle has a unique Steiner inellipse which is interior to the triangle and tangent at the midpoints of the sides. Marden's theorem shows how to find the foci of this ellipse.^[34] This ellipse has the greatest area of any ellipse tangent to all three sides of the triangle.

The Mandart inellipse of a triangle is the ellipse inscribed within the triangle tangent to its sides at the contact points of its excircles.

For any ellipse inscribed in a triangle ABC, let the foci be P and Q. Then^[35]

${\displaystyle {\frac {{\overline {PA}}\cdot {\overline {QA}}}{{\overline {CA}}\cdot {\overline {AB}}}}+{\frac {{\overline {PB}}\cdot {\overline {QB}}}{{\overline {AB}}\cdot {\overline {BC}}}}+{\frac {{\overline {PC}}\cdot {\overline {QC}}}{{\overline {BC}}\cdot {\overline {CA}}}}=1.}$

Convex polygon

Every convex polygon with area T can be inscribed in a triangle of area at most equal to 2T. Equality holds (exclusively) for a parallelogram.^[36]

Hexagon

The Lemoine hexagon is a cyclic hexagon with vertices given by the six intersections of the sides of a triangle with the three lines that are parallel to the sides and that pass through its symmedian point. In either its simple form or its self-intersecting form, the Lemoine hexagon is interior to the triangle with two vertices on each side of the triangle.

Squares

Every acute triangle has three inscribed squares (squares in its interior such that all four of a square's vertices lie on a side of the triangle, so two of them lie on the same side and hence one side of the square coincides with part of a side of the triangle). In a right triangle two of the squares coincide and have a vertex at the triangle's right angle, so a right triangle has only two distinct inscribed squares. An obtuse triangle has only one inscribed square, with a side coinciding with part of the triangle's longest side. Within a given triangle, a longer common side is associated with a smaller inscribed square. If an inscribed square has side of length q_a and the triangle has a side of length a, part of which side coincides with a side of the square, then q_a, a, the altitude h_a from the side a, and the triangle's area T are related according to^[37][38]

${\displaystyle q_{a}={\frac {2Ta}{a^{2}+2T}}={\frac {ah_{a}}{a+h_{a}}}.}$

The largest possible ratio of the area of the inscribed square to the area of the triangle is 1/2, which occurs when a² = 2T, q = a/2, and the altitude of the triangle from the base of length a is equal to a. The smallest possible ratio of the side of one inscribed square to the side of another in the same non-obtuse triangle is ${\displaystyle 2{\sqrt {2}}/3=0.94....}$^[38] Both of these extreme cases occur for the isosceles right triangle.

Triangles

From an interior point in a reference triangle, the nearest points on the three sides serve as the vertices of the pedal triangle of that point. If the interior point is the circumcenter of the reference triangle, the vertices of the pedal triangle are the midpoints of the reference triangle's sides, and so the pedal triangle is called the midpoint triangle or medial triangle. The midpoint triangle subdivides the reference triangle into four congruent triangles which are similar to the reference triangle.

The Gergonne triangle or intouch triangle of a reference triangle has its vertices at the three points of tangency of the reference triangle's sides with its incircle. The extouch triangle of a reference triangle has its vertices at the points of tangency of the reference triangle's excircles with its sides (not extended).

Figures circumscribed about a triangle

The tangential triangle of a reference triangle (other than a right triangle) is the triangle whose sides are on the tangent lines to the reference triangle's circumcircle at its vertices.

As mentioned above, every triangle has a unique circumcircle, a circle passing through all three vertices, whose center is the intersection of the perpendicular bisectors of the triangle's sides.

Further, every triangle has a unique Steiner circumellipse, which passes through the triangle's vertices and has its center at the triangle's centroid. Of all ellipses going through the triangle's vertices, it has the smallest area.

The Kiepert hyperbola is the unique conic which passes through the triangle's three vertices, its centroid, and its circumcenter.

Of all triangles contained in a given convex polygon, there exists a triangle with maximal area whose vertices are all vertices of the given polygon.^[39]

Specifying the location of a point in a triangle

One way to identify locations of points in (or outside) a triangle is to place the triangle in an arbitrary location and orientation in the Cartesian plane, and to use Cartesian coordinates. While convenient for many purposes, this approach has the disadvantage of all points' coordinate values being dependent on the arbitrary placement in the plane.

Two systems avoid that feature, so that the coordinates of a point are not affected by moving the triangle, rotating it, or reflecting it as in a mirror, any of which give a congruent triangle, or even by rescaling it to give a similar triangle:

• Trilinear coordinates specify the relative distances of a point from the sides, so that coordinates ${\displaystyle x:y:z}$ indicate that the ratio of the distance of the point from the first side to its distance from the second side is ${\displaystyle x:y}$ , etc.
• Barycentric coordinates of the form ${\displaystyle \alpha :\beta :\gamma }$ specify the point's location by the relative weights that would have to be put on the three vertices in order to balance the otherwise weightless triangle on the given point.

Non-planar triangles

A non-planar triangle is a triangle which is not contained in a (flat) plane. Some examples of non-planar triangles in non-Euclidean geometries are spherical triangles in spherical geometry and hyperbolic triangles in hyperbolic geometry.

While the measures of the internal angles in planar triangles always sum to 180°, a hyperbolic triangle has measures of angles that sum to less than 180°, and a spherical triangle has measures of angles that sum to more than 180°. A hyperbolic triangle can be obtained by drawing on a negatively curved surface, such as a saddle surface, and a spherical triangle can be obtained by drawing on a positively curved surface such as a sphere. Thus, if one draws a giant triangle on the surface of the Earth, one will find that the sum of the measures of its angles is greater than 180°; in fact it will be between 180° and 540°.^[40] In particular it is possible to draw a triangle on a sphere such that the measure of each of its internal angles is equal to 90°, adding up to a total of 270°.

Specifically, on a sphere the sum of the angles of a triangle is

180° × (1 + 4f),

where f is the fraction of the sphere's area which is enclosed by the triangle. For example, suppose that we draw a triangle on the Earth's surface with vertices at the North Pole, at a point on the equator at 0° longitude, and a point on the equator at 90° West longitude. The great circle line between the latter two points is the equator, and the great circle line between either of those points and the North Pole is a line of longitude; so there are right angles at the two points on the equator. Moreover, the angle at the North Pole is also 90° because the other two vertices differ by 90° of longitude. So the sum of the angles in this triangle is 90° + 90° + 90° = 270°. The triangle encloses 1/4 of the northern hemisphere (90°/360° as viewed from the North Pole) and therefore 1/8 of the Earth's surface, so in the formula f = 1/8; thus the formula correctly gives the sum of the triangle's angles as 270°.

From the above angle sum formula we can also see that the Earth's surface is locally flat: If we draw an arbitrarily small triangle in the neighborhood of one point on the Earth's surface, the fraction f of the Earth's surface which is enclosed by the triangle will be arbitrarily close to zero. In this case the angle sum formula simplifies to 180°, which we know is what Euclidean geometry tells us for triangles on a flat surface.

Triangles in construction

Main article: Truss

The Flatiron Building in New York is shaped like a triangular prism

Rectangles have been the most popular and common geometric form for buildings since the shape is easy to stack and organize; as a standard, it is easy to design furniture and fixtures to fit inside rectangularly shaped buildings. But triangles, while more difficult to use conceptually, provide a great deal of strength. As computer technology helps architects design creative new buildings, triangular shapes are becoming increasingly prevalent as parts of buildings and as the primary shape for some types of skyscrapers as well as building materials. In Tokyo in 1989, architects had wondered whether it was possible to build a 500-story tower to provide affordable office space for this densely packed city, but with the danger to buildings from earthquakes, architects considered that a triangular shape would be necessary if such a building were to be built.^[41]

In New York City, as Broadway crisscrosses major avenues, the resulting blocks are cut like triangles, and buildings have been built on these shapes; one such building is the triangularly shaped Flatiron Building which real estate people admit has a "warren of awkward spaces that do not easily accommodate modern office furniture" but that has not prevented the structure from becoming a landmark icon.^[42] Designers have made houses in Norway using triangular themes.^[43] Triangle shapes have appeared in churches^[44] as well as public buildings including colleges^[45] as well as supports for innovative home designs.^[46]

Triangles are sturdy; while a rectangle can collapse into a parallelogram from pressure to one of its points, triangles have a natural strength which supports structures against lateral pressures. A triangle will not change shape unless its sides are bent or extended or broken or if its joints break; in essence, each of the three sides supports the other two. A rectangle, in contrast, is more dependent on the strength of its joints in a structural sense. Some innovative designers have proposed making bricks not out of rectangles, but with triangular shapes which can be combined in three dimensions.^[47] It is likely that triangles will be used increasingly in new ways as architecture increases in complexity. It is important to remember that triangles are strong in terms of rigidity, but while packed in a tessellating arrangement triangles are not as strong as hexagons under compression (hence the prevalence of hexagonal forms in nature). Tessellated triangles still maintain superior strength for cantilevering however, and this is the basis for one of the strongest man made structures, the tetrahedral truss.

See also

Apollonius' theorem Congruence (geometry) Desargues' theorem Dragon's Eye (symbol) Fermat point Hadwiger–Finsler inequality Heronian triangle

Integer triangle Law of cosines Law of sines Law of tangents Lester's theorem List of triangle inequalities List of triangle topics Ono's inequality

Pedal triangle Pedoe's inequality Pythagorean theorem Special right triangles Triangle center Triangular number Triangulated category Triangulation (topology)

Notes

1. Euclid defines isosceles triangles based on the number of equal sides, i.e. only two equal sides. An alternative approach defines isosceles triangles based on shared properties, i.e. equilateral triangles are a special case of isosceles triangles. wikt:Isosceles triangle
2. The n external angles of any n-sided convex polygon add up to 360 degrees.
3. Again, in all cases "mirror images" are also similar.
4. All pairs of congruent triangles are also similar; but not all pairs of similar triangles are congruent.

References

1. "List of Geometry and Trigonometry Symbols". Math Vault. 17 April 2020. Retrieved 1 September 2020.
2. Weisstein, Eric W. "Triangle". MathWorld.
3. "Triangles - Equilateral, Isosceles and Scalene". www.mathsisfun.com. Retrieved 1 September 2020.
4. Euclid Elements Book I Definition 20
5. Weisstein, Eric W. "Equilateral Triangle". MathWorld.
6. Weisstein, Eric W. "Isosceles Triangle". MathWorld.
7. Weisstein, Eric W. "Scalene triangle". MathWorld.
8. Zeidler, Eberhard (2004). Oxford Users' Guide to Mathematics. Oxford University Press. p. 729. ISBN 978-0-19-850763-5.
9. "Euclid's Elements, Book I, Proposition 32".
10. Vardan Verdiyan & Daniel Campos Salas, "Simple trigonometric substitutions with broad results", Mathematical Reflections no 6, 2007.
11. Longuet-Higgins, Michael S., "On the ratio of the inradius to the circumradius of a triangle", Mathematical Gazette 87, March 2003, 119–120.
12. Prof. David E. Joyce. "The Laws of Cosines and Sines". Clark University. Retrieved 1 November 2008.
13. Weisstein, Eric W. "Law of Tangents". Wolfram MathWorld. Retrieved 26 July 2012.
14. The Āryabhaṭīya by Āryabhaṭa (translated into English by Walter Eugene Clark, 1930) hosted online by the Internet Archive.
15. Weisstein, Eric W. "Triangle area". MathWorld.
16. Bart Braden (1986). "The Surveyor's Area Formula" (PDF). The College Mathematics Journal. 17 (4): 326–337. doi:10.2307/2686282. JSTOR 2686282.
17. Benyi, Arpad, "A Heron-type formula for the triangle," Mathematical Gazette" 87, July 2003, 324–326.
18. Mitchell, Douglas W., "A Heron-type formula for the reciprocal area of a triangle," Mathematical Gazette 89, November 2005, 494.
19. Mitchell, Douglas W., "A Heron-type area formula in terms of sines," Mathematical Gazette 93, March 2009, 108–109.
20. Sa ́ndor Nagydobai Kiss, "A Distance Property of the Feuerbach Point and Its Extension", Forum Geometricorum 16, 2016, 283–290.
21. "Circumradius". AoPSWiki. Archived from the original on 20 June 2013. Retrieved 26 July 2012.
22. Mitchell, Douglas W., "The area of a quadrilateral," Mathematical Gazette 93, July 2009, 306–309.
23. Pathan, Alex, and Tony Collyer, "Area properties of triangles revisited," Mathematical Gazette 89, November 2005, 495–497.
24. Baker, Marcus, "A collection of formulae for the area of a plane triangle," Annals of Mathematics, part 1 in vol. 1(6), January 1885, 134–138; part 2 in vol. 2(1), September 1885, 11–18. The formulas given here are #9, #39a, #39b, #42, and #49. The reader is advised that several of the formulas in this source are not correct.
25. Chakerian, G.D. "A Distorted View of Geometry." Ch. 7 in Mathematical Plums (R. Honsberger, editor). Washington, DC: Mathematical Association of America, 1979: 147.
26. Rosenberg, Steven; Spillane, Michael; and Wulf, Daniel B. "Heron triangles and moduli spaces", Mathematics Teacher 101, May 2008, 656–663.
27. Posamentier, Alfred S., and Lehmann, Ingmar, The Secrets of Triangles, Prometheus Books, 2012.
28. Dunn, J.A., and Pretty, J.E., "Halving a triangle," Mathematical Gazette 56, May 1972, 105–108.
29. Altshiller-Court, Nathan, College Geometry, Dover, 2007.
30. Oxman, Victor. "On the existence of triangles with given lengths of one side and two adjacent angle bisectors", Forum Geometricorum 4, 2004, 215–218.
31. Mitchell, Douglas W. (2013), "Perpendicular Bisectors of Triangle Sides", Forum Geometricorum 13, 53-59.
32. Johnson, Roger A., Advanced Euclidean Geometry, Dover Publ. Co., 2007
33. Altshiller-Court (1925, pp. 70–71) harvtxt error: no target: CITEREFAltshiller-Court1925 (help)
34. Kalman, Dan. "An Elementary Proof of Marden's Theorem", 2008, American Mathematical Monthly 115, 330–338.
35. Allaire, Patricia R.; Zhou, Junmin; and Yao, Haishen, "Proving a nineteenth century ellipse identity", Mathematical Gazette 96, March 2012, 161–165.
36. Weisstein, Eric W. "Triangle Circumscribing". Wolfram Math World.
37. Bailey, Herbert, and DeTemple, Duane, "Squares inscribed in angles and triangles", Mathematics Magazine 71(4), 1998, 278–284.
38. Victor Oxman and Moshe Stupel, "Why Are the Side Lengths of the Squares Inscribed in a Triangle so Close to Each Other?", Forum Geometricorum 13 (2013) 113–115.
39. -, Christos. "Is the area of intersection of convex polygons always convex?". Math Stack Exchange.
40. Watkins, Matthew, Useful Mathematical and Physical Formulae, Walker and Co., 2000.
41. Associated Press (10 November 1989). "Tokyo Designers Envision 500-Story Tower". Los Angeles Times. Retrieved 5 March 2011. A construction company said Thursday that it has designed a 500-story skyscraper for Tokyo, ... The building is shaped like a triangle, becoming smaller at the top to help it absorb shock waves. It would have a number of tunnels to let typhoon winds pass through rather than hitting the building with full force.
42. Stapinski, Helene (26 May 2010). "A Quirky Building That Has Charmed Its Tenants". The New York Times. Retrieved 5 March 2011. Though it is hard to configure office space in a triangle
43. Jodidio, Philip (2009). "Triangle House in Norway". Architecture Week. Retrieved 5 March 2011. Local zoning restrictions determined both the plan and the height of the Triangle House in Nesodden, Norway, which offers views toward the sea through a surrounding pine forest.
44. Metz, Tracy (July 2009). "The Chapel of the Deaconesses of Reuilly". Architectural Record. Retrieved 5 March 2011. the classical functions of a church in two pure forms: a stark triangle of glass and, inside it, a rounded, egglike structure made of wood.
45. Deborah Snoonian, P.E. (5 March 2011). "Tech Briefs: Seismic framing technology and smart siting aid a California community college". Architectural Record. Retrieved 5 March 2011. More strength, less material ... They share a common material language of structural steel, glass and metal panels, and stucco cladding; their angular, dynamic volumes, folded roof plates, and triangular forms are meant to suggest the plate tectonics of the shifting ground planes they sit on.
46. Sarah Amelar (November 2006). "Prairie Ridge Ecostation for Wildlife and Learning". Architectural Record. Retrieved 5 March 2011. Perched like a tree house, the $300,000 structure sits lightly on the terrain, letting the land flow beneath it. Much of the building rests on three triangular heavy-timber frames on a concrete pad.
47. Joshua Rothman (13 March 2011). "Building a better brick". Boston Globe. Retrieved 5 March 2011. Bricks are among the world’s oldest building materials — the first were used as long ago as 7,500 B.C. ... An especially beautiful proposal by Rizal Muslimin at the Massachusetts Institute of Technology came in as a runner-up: BeadBricks are flat, triangular bricks that can be combined in three dimensions (rather than the usual two).

External links

Wikimedia Commons has media related to Triangles.

Look up triangle in Wiktionary, the free dictionary.

• Ivanov, A.B. (2001) [1994], "Triangle", Encyclopedia of Mathematics, EMS Press
• Clark Kimberling: Encyclopedia of triangle centers. Lists some 5200 interesting points associated with any triangle.